Premium Only Content
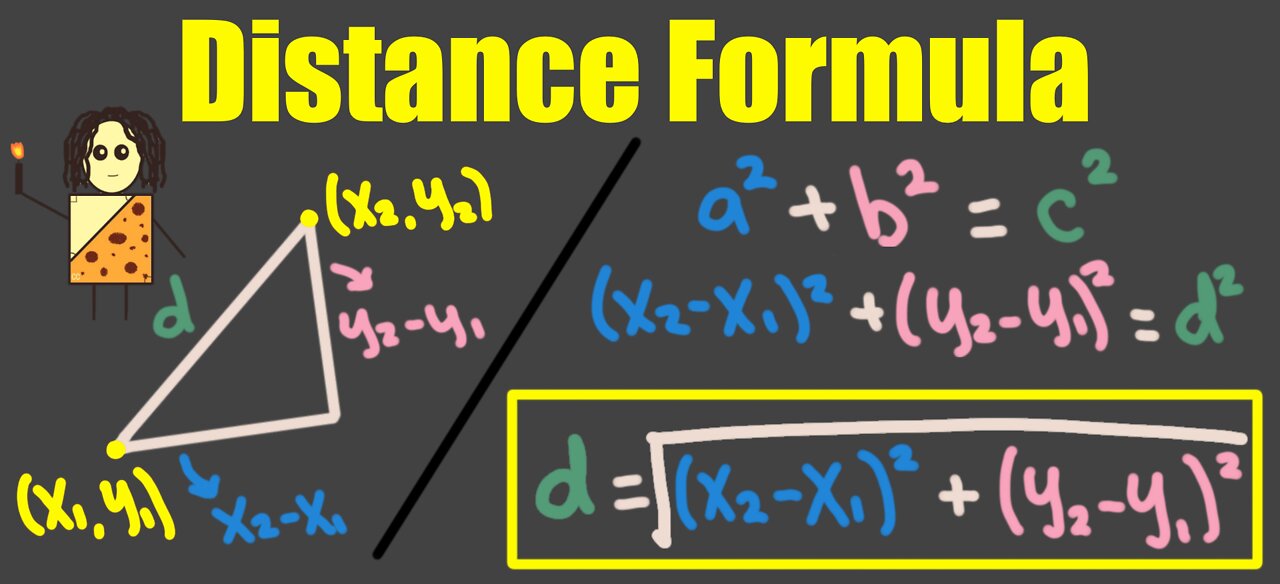
Deriving and Using the Distance Formula
Hello! For today's video, I will talk about the distance formula. In math, there are just an endless amount of formulas that we should memorize but sometimes the best way to memorize the formula is to understand where it comes from. One of the most important formulas that you should remember is the Pythagorean Theorem.
Pythagorean Theorem: a^2 + b^2 = c^2
Well, would you believe me if I said the distance formula is actually derived from the Pythagorean Theorem.
Distance Formula: d = sqrt [(x2-x1)^2 + (y2-y1)^2]
The formula looks quite different for now but in the first half of the video, I show you how the distance formula is derived from the pythagorean theorem. In a right triangle, we have each side represented by a, b, and c. The distance between two points can always be represented by a right triangle where the hypotenuse c would be the distance we are looking for. a would be represented as the horizontal difference between the two points (x2-x1) and b would be represented as the vertical difference between the two points (y2-y1). Anyways, let's take a look at the video!
Sections in the video:
0:00 Introduction to the Distance Formula
2:03 Deriving the Distance Formula from the Pythagorean Theorem
8:30 Practice Problem #1
10:45 Practice Problem #2
12:37 Practice Problem #3
Thanks for watching! Please drop a like and subscribe to my channel.Don’t forget to let me know if you have any questions in the comments below.
For more math videos like this, be sure to subscribe to my channel https://rumble.com/user/CavemanChang
Hope you enjoyed my video! Thanks for watching!
Credits:
Custom Titles: Simple Video Making
Link: https://www.youtube.com/channel/UCOsPt0xImHyuRGU7BntSqDQ
-
 12:42
12:42
Learn Math with Caveman Chang
2 years agoProperties of Modular Arithmetic
18 -
 59:31
59:31
Squaring The Circle, A Randall Carlson Podcast
8 hours ago#039: How Politics & War, Art & Science Shape Our World; A Cultural Commentary From Randall Carlson
1.11K2 -
 13:21
13:21
Misha Petrov
8 hours agoThe CRINGIEST Thing I Have Ever Seen…
1.73K22 -
 11:45
11:45
BIG NEM
4 hours agoWe Blind Taste Tested the Best Jollof in Toronto 🇳🇬🇬🇭
28 -
 15:40
15:40
Fit'n Fire
7 hours agoArsenal SLR106f & LiteRaider AK Handguard from 1791 Industries
881 -
 8:34
8:34
Mike Rowe
6 days agoWhat You Didn't Hear At Pete's Confirmation Hearing | The Way I Heard It with Mike Rowe
33.1K18 -
 7:13:44
7:13:44
TonYGaMinG
9 hours ago🟢LATEST! KINGDOM COME DELIVERANCE 2 / NEW EMOTES / BLERPS #RumbleGaming
56.7K4 -
 40:17
40:17
SLS - Street League Skateboarding
4 days agoEVERY 9 CLUB IN FLORIDA! Looking back at SLS Jacksonville 2021 & 2022 - Yuto, Jagger, Sora & more...
101K1 -
 2:00:47
2:00:47
PaddysParlorGames
18 hours agoSunday Parlor Chill: GOBSTEIN
56.1K3 -
 LIVE
LIVE
Major League Fishing
5 days agoLIVE! - Bass Pro Tour: Stage 2 - Day 4
145 watching