Premium Only Content
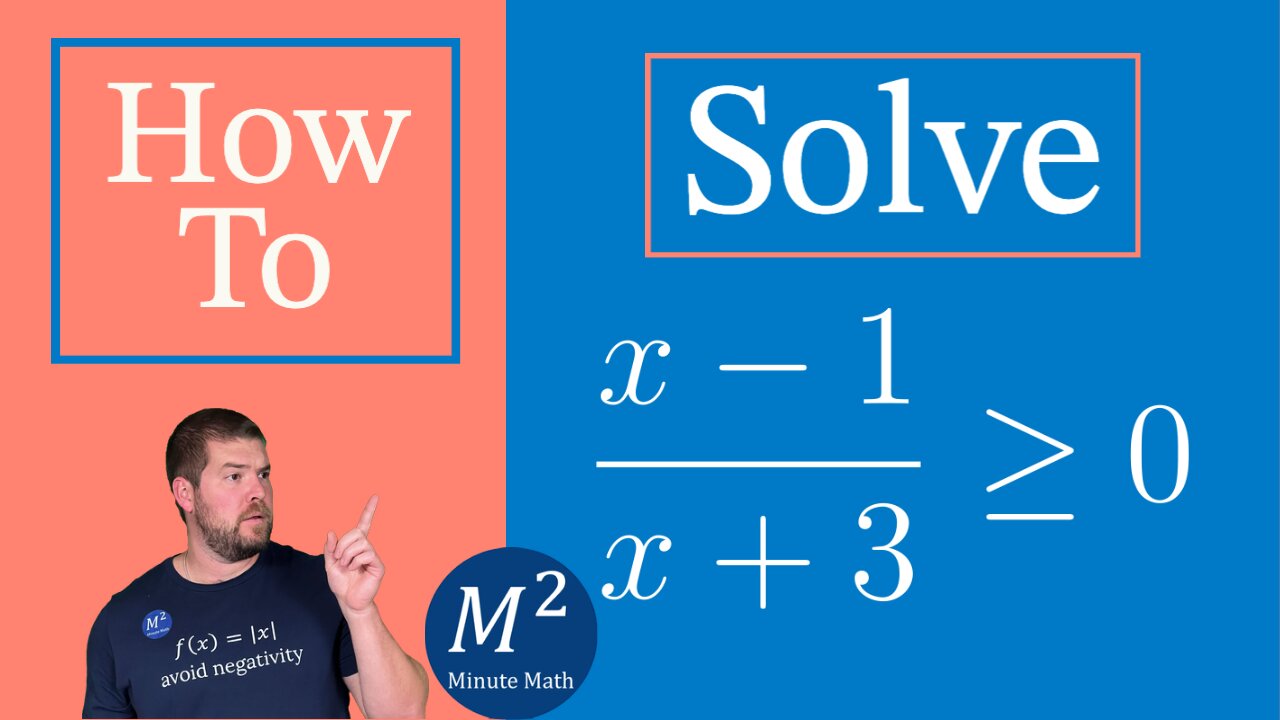
Solve a Rational Inequality | Solve (x-1)/(x+3) ≥ 0 and write the solution in interval notation
In this video we learn how to Solve a Rational Inequality. We solve (x-1)/(x+3) ≥ 0 and write the solution in interval notation.
The steps we follow on how to solve a rational inequality are:
1) Write the inequality as one quotient on the left and zero on the right.
2) Determine the critical points–the points where the rational expression will be zero or undefined.
3) Use the critical points to divide the number line into intervals.
4) Test a value in each interval. Above the number line show the sign of each factor of the numerator and denominator in each interval. Below the number line show the sign of the quotient.
5) Determine the intervals where the inequality is correct. Write the solution in interval notation.
#rationalinequalities #inequalities #minutemath #algebrahelp #algebra2 #intermediatealgebra #mathhelp #onlinemathvideos #math #mathvideos #oer #openeducation #freemathvideos
Learn more on this topic:
https://minutemath.com/algebra-2/solve-rational-inequalities/
Visit our website Math Help and Math Merch:
https://minutemath.com/
Follow us for...
Tweets: https://twitter.com/minutemath
Instagram: https://www.instagram.com/minutemath/
TikTok: https://www.tiktok.com/@therealminutemath
Facebook: https://www.facebook.com/MinuteMath/
Personal Instagram: https://www.instagram.com/gannonforpresident/
Business Instagram: https://www.instagram.com/minutebusinessacademy/
CC Licensed Content, Original
Revision and Adaption. Provided by: Minute Math. License: CC BY 4.0
CC Licensed Content, Shared Previously
Marecek, L., & Mathis, A. H. (2020). Multiply and Divide Rational Expressions. In Intermediate Algebra 2e. OpenStax. https://openstax.org/books/intermediate-algebra-2e/pages/7-6-solve-rational-inequalities. License: CC BY 4.0. Access for free at https://openstax.org/books/intermediate-algebra-2e/pages/1-introduction
-
 2:18
2:18
Minute Math
9 months agoHow many possible Circuits in a Complete Graph? | Graph Theory Basics
921 -
 7:49
7:49
BOOK&TABLE Clips
4 years agoInequalities | Compound Inequality | Interval Notation
15 -
 2:55
2:55
Minute Math
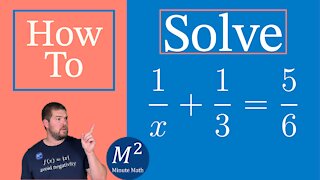
3 years ago $0.02 earnedHow to Solve Rational Equations | Solve 1/x+1/3=5/6 | Minute Math
40 -
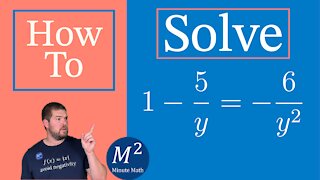 3:17
3:17
Minute Math
3 years ago $0.01 earnedHow to Solve Rational Equations | Solve 1-5/y=-6/y² | Minute Math
46 -
 4:31
4:31
Minute Math
3 years agoHow to Solve a Compound Inequality with "and" (No Solution Example) | Part 3 of 3 | Minute Math
33 -
 3:44
3:44
Minute Math
3 years agoHow to Solve a Rational Equation for a Specific Variable | Solve 1/c+1/m=1 for c | Minute Math
23 -
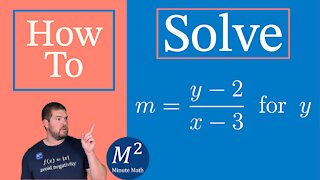 2:44
2:44
Minute Math
3 years agoHow to Solve a Rational Equation for a Specific Variable | Solve m=(y-2)/(x-3) for y | Minute Math
27 -
 4:18
4:18
Minute Math
3 years agoHow to Solve a Compound Inequality with "or" | Part 2 of 2 | Minute Math
32 -
 4:07
4:07
Minute Math
3 years agoHow to Solve a Compound Inequality with "or" | Part 1 of 2 | Minute Math
23 -
 4:29
4:29
Minute Math
3 years agoHow to Solve a Compound Inequality with "and" | Part 2 of 3 | Minute Math
46