Premium Only Content
Solving quadratic equations - Factoring - Discriminant - IntoMath
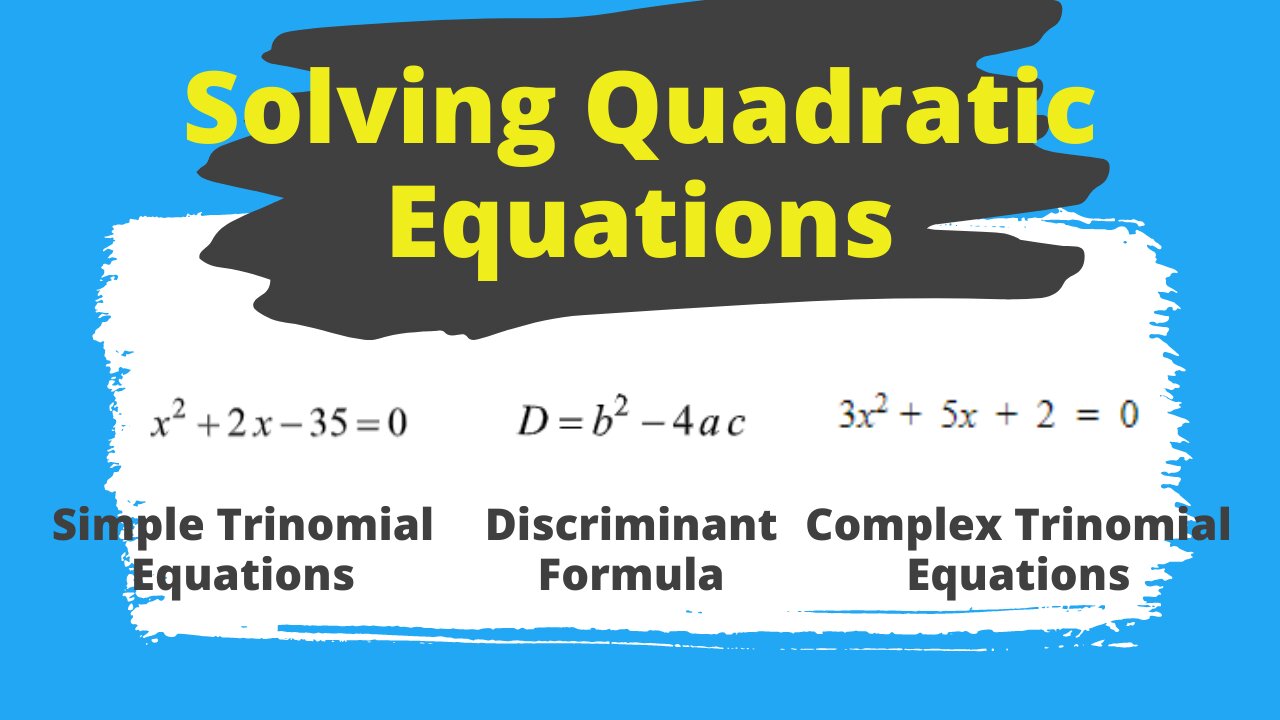
Solving quadratic equations requires a good understanding of and proficiency with a number of concepts. A quadratic equation is an equation of the form ax2 + bx + c = 0. This is a standard form equation. A quadratic equation can also be recorded in the factored form a(x – r)(x – s) = 0, where r and s are the roots of the equation.
In order to determine the solutions (roots or x-intercepts of the parabola) of the quadratic equation and when the equation is given in a standard form, we can either try to factor the equation or use the Quadratic Formula.
The method of solving quadratic equations by factoring depends whether the standard form equation is given as a simple or as a complex trinomial and whether the factors could be easily identified.
If the a value of the equation is a = 1 and the equation is x2 + bx + c = 0, then x1 + x2 = -b and (x1)(x2) = c
However, if a is not 0 or 1, then the equation could be solved by factoring using the decomposition method (watch the video lesson to see how).
Sometimes factors are not very “nice” numbers. In such cases it is difficult to factor and the Quadratic Formula should be used instead.
Before setting up the Quadratic Formula it is always a good idea to check whether the equation is at all solvable. To check how many possible solutions the quadratic equation has we use the Discriminant.
The Discriminant is an expression that is part of the Quadratic Formula. The note above contains all possible scenarios for when the Discriminant is positive, negative or 0.
Once the quadratic equation has been solved, the solutions can be used to graph the quadratic function or a statement based on the real life problem could be made. For example, if we are talking about a kicked ball, then the x-intercepts represent the time when the ball touches the ground as it was kicked and when it landed.
More free math help and activities: intomath.org
-
 5:28
5:28
IntoMath Grade 9 Lessons
3 years ago $0.63 earnedSolving equations with brackets - IntoMath
1.16K -
 8:01
8:01
IntoMath Grade 8 Lessons
3 years ago $0.20 earnedSolving linear equations - Pythagorean theorem - IntoMath
666 -
 7:16
7:16
IntoMath Grade 7 Lessons
3 years ago $0.14 earnedCollecting like terms - Solving linear equations - IntoMath
369 -
 15:57
15:57
IntoMath Grade 10 Lessons
3 years ago $0.01 earnedSolving the system of two linear equations - IntoMath
271 -
 3:32
3:32
IntoMath Grade 9 Lessons
3 years ago $0.59 earnedDistributive property - Common factoring - IntoMath
1.13K -
 13:15
13:15
IntoMath Grade 10 Lessons
3 years ago $0.01 earnedQuadratic function and its graph explained - IntoMath
176 -
 4:56
4:56
IntoMath Grade 8 Lessons
3 years ago $0.46 earnedProportions - IntoMath
1K -
 4:48
4:48
IntoMath Grade 6 Lessons
3 years ago $0.04 earnedDecimals - Place value - IntoMath
2841 -
 10:23
10:23
IntoMath Grade 10 Lessons
3 years agoSimplifying expressions with exponents - IntoMath
70 -
 4:45
4:45
IntoMath Grade 7 Lessons
3 years ago $0.09 earnedMultiplying and Dividing Fractions - IntoMath
608