Premium Only Content
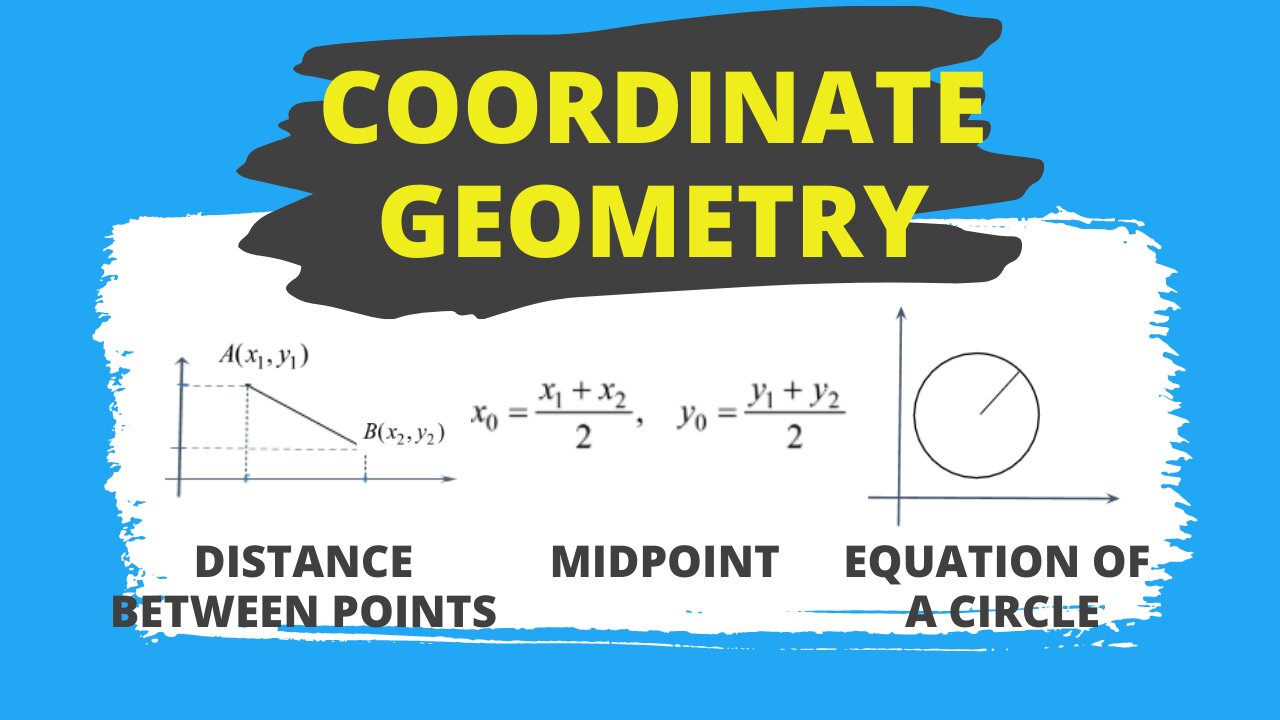
Distance between two points - Midpoint - Equation of circle - IntoMath
Coordinate geometry helps us find the distance between the points, determine the side lengths and angles of geometric shapes using a Coordinate Plane.
There is a number of ways to do that and it is really fun to do when you understand why we take certain steps.
In coordinate geometry you need to know some terminology. For example, you need to know that the word “origin” means a point with coordinates (0,0). Or that the word “subscript” means a small letter or number below a regular sized one (E-g.: x2).
This lesson demonstrates how to determine the distance between the two points using their coordinates. It also explains how to find the midpoint on a line segment.
In this lesson we are also looking at the equation of a circle.
The general equation of a circle with the center not at the origin is
(x – a)2 + (y – b)2 = r2
where r is the radius of a circle and a,b are not 0.
When the center of a circle is at the origin, then a and b are equal to 0.
In general, coordinate geometry is a fascinating branch of mathematics. It is like learning to read a mathematical map.
Did you know that any pdf file on the web is an example of a coordinate geometry? The words and symbols in these files are written using the coordinate geometry. If a document contains text and images, they are placed according to a Coordinate Plane using the concepts of a distance between two points, slope of a line, as well as trigonometry.
Photo editing software are based on coordinate geometry. Changing the dimensions of an image or moving it around the screen – everything is based on the concept and the position of every point has its coordinates (x,y).
In a GPS system that we use to get from one place to another, the longitude and the latitude of a place are its coordinates.
More free math help and activities: intomath.org
-
 2:09
2:09
KTNV
4 years agoNew technology tracks distance between workers
9 -
 2:25
2:25
KJRH
4 years agoTPS parents decide between virtual academy and distance learning
30 -
 0:28
0:28
World around us
4 years agoThe fight between the two drakes
335 -
 2:40
2:40
DRWarren62
3 years agoMaking a circle.
307 -
 2:14
2:14
KMGH
4 years agoStrip club owner gets creative to skirt 25-foot distance required between dancer and customers
42 -
 0:16
0:16
AbbyG
4 years ago $0.07 earnedHope between the raindrops
238 -
 0:34
0:34
Buzzvideos - EN
4 years agoDog stuck between two parked cars
29 -
 0:34
0:34
Buzzvideos - EN
4 years agoDog stuck between two parked cars
19 -
 0:34
0:34
Buzzvideos - EN
4 years agoViolent argument between two drivers
42 -
 1:29
1:29
Buzzvideos - EN
4 years agoVideo shows unprecedented conversation between two cats
135