Premium Only Content
BHASKARA FORMULA: Exercise 12.4
The Bhaskara formula, also known as the quadratic formula, is a mathematical formula that is used to solve quadratic equations of the ax² + bx + c = 0 form, where A, B and C are constant.
Bhaskara formula
Bhaskara's formula is:
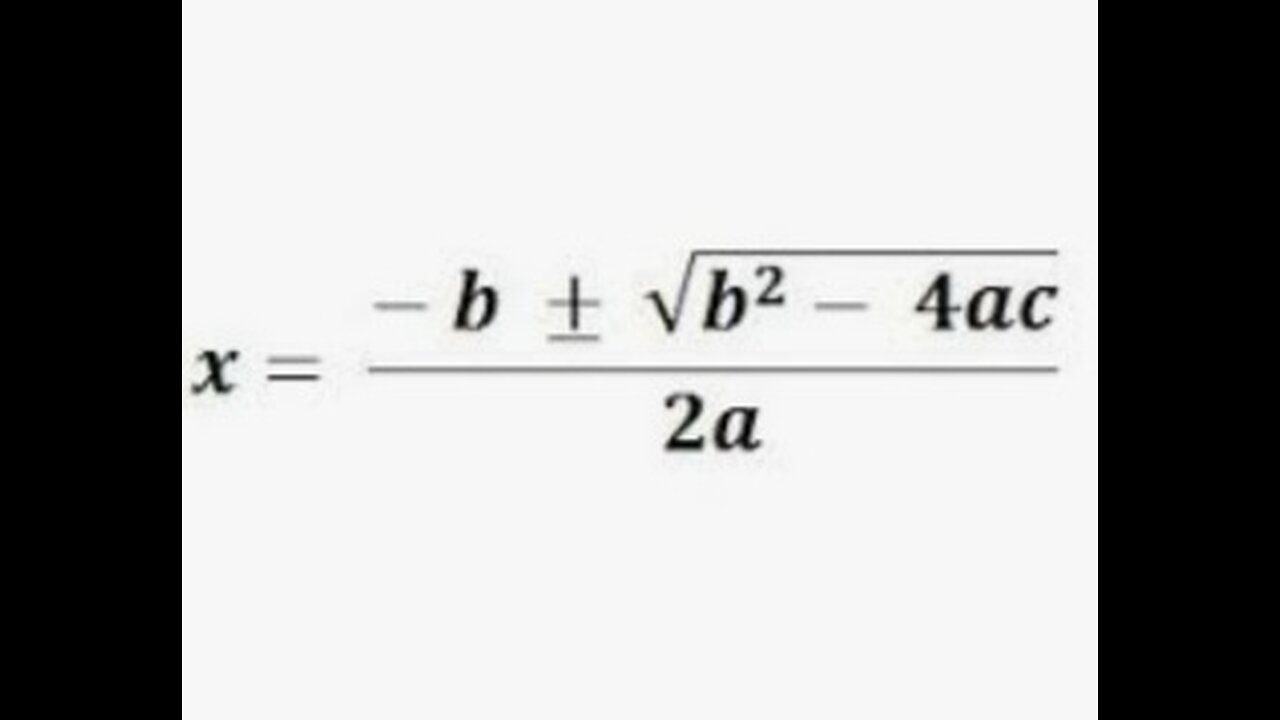
x = (-b ± √ (b² - 4ac)) / 2a
Where:
- X is the solution of the equation
- A is the coefficient of x²
- B is the coefficient of x
- C is the constant term
- √ It is the square root symbol
History
Bhaskara's formula was discovered by Indian mathematician Bhaskara in the twelfth century. However, the formula was also discovered independently by other mathematicians, such as Greek Euclid and Persian al-Khwarizmi.
Example
Suppose we want to solve the equation x² + 5x + 6 = 0. Using the Bhaskara formula, we get:
x = (-5 ± √ (5² - 4 (1) (6))) / 2 (1)
x = (-5 ± √ (25 - 24)) / 2
x = (-5 ± √1) / 2
x = (-5 ± 1) / 2
Therefore, the solutions of the equation are x = -2 and x = -3.
Importance
The Bhaskara formula is a fundamental tool in algebra and is used in a wide variety of applications, such as the resolution of quadratic equations, polynomial factorization and the resolution of optimization problems.
-
 8:21
8:21
DEADBUGsays
21 hours agoThe Crossbow Killer
1.01K3 -
 8:40
8:40
Tundra Tactical
16 hours ago $0.03 earnedThe Executive Order Wishlist.
564 -
 7:22:52
7:22:52
SpartakusLIVE
15 hours agoSaturday SPARTOON Solos to Start || Duos w/ StevieT Later
90.2K2 -
 28:40
28:40
SLS - Street League Skateboarding
8 days agoTOP MOMENTS IN WOMEN’S SLS HISTORY! ALL THE 9’s - Rayssa Leal, Leticia Bufoni, Chloe Covell & more…
39.7K11 -
 2:03:03
2:03:03
The Connect: With Johnny Mitchell
13 hours agoHow Mexican & Chinese Cartels Control Illegal Marijuana Cultivation In America Using SLAVE Labor
1231 -
 14:46
14:46
Mrgunsngear
14 hours ago $0.63 earnedPrimary Arms GLx 1x Prism With ACSS Reticle Review
3.12K5 -
 22:37
22:37
Degenerate Plays
13 hours agoI'm A Psychic Now - Elden Ring : Part 73
107 -
 2:32:02
2:32:02
Jamie Kennedy
7 hours agoEp. 195 Horror Legend Barbara Crampton
89 -
 23:00
23:00
Exploring With Nug
1 day ago $32.38 earnedHis Truck Was Found Crashed in the Woods… But He’s Gone!
123K8 -
 27:09
27:09
MYLUNCHBREAK CHANNEL PAGE
1 day agoDilmun: Where Life Never Ends
91.5K56