Premium Only Content
This video is only available to Rumble Premium subscribers. Subscribe to
enjoy exclusive content and ad-free viewing.
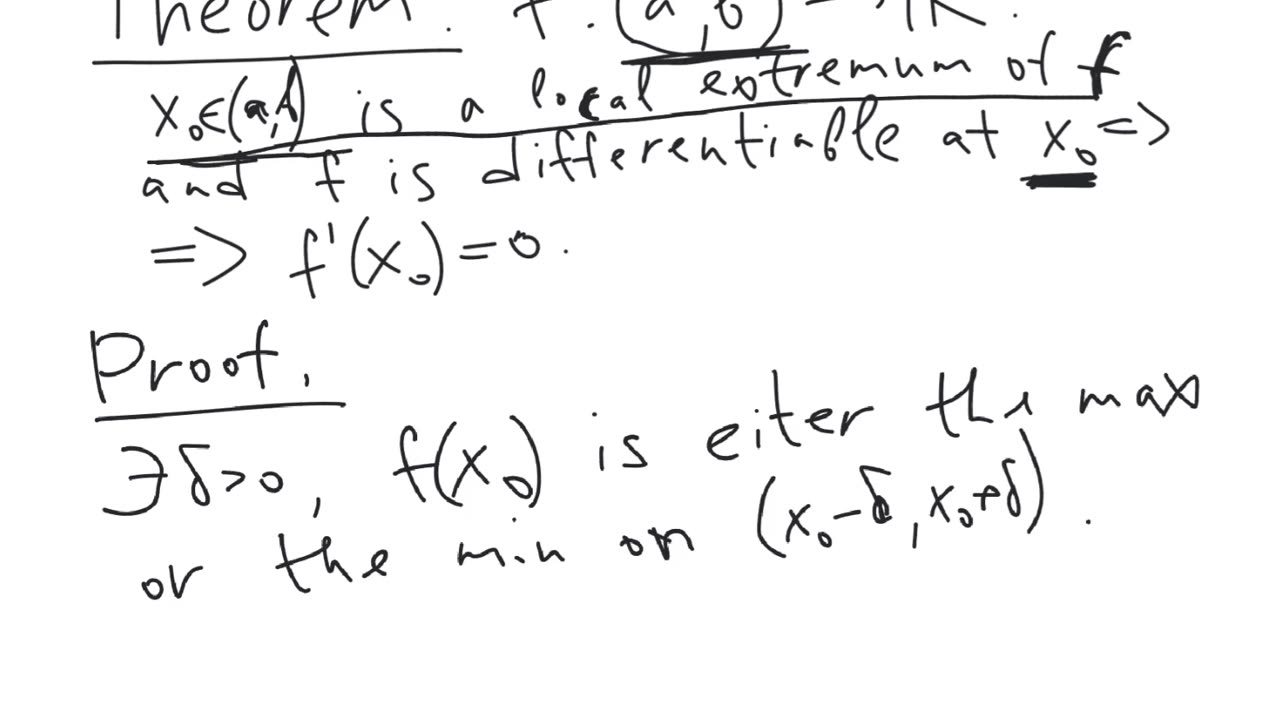
Fermat's theorem (stationary point theorem, interior extremum theorem) | math analysis | elementary
5 months ago
14
Science
mathematics
analysis
mathematical analysis
real analysis
calculus
derivative
differentiable
function
extremum
local extremum
Episode 77.
Fermat's theorem (stationary point theorem, interior extremum theorem) | math analysis | elementary.
Fermat's theorem (stationary point theorem, interior extremum theorem) | mathematical analysis | elementary level.
Branch of mathematics: mathematical analysis.
Difficulty level: elementary.
Fermat's theorem (stationary point theorem, interior extremum theorem). Let $f$ be a function defined on an interval (a,b). Suppose that it has a local extremum at an interior point $x_0 \in (a,b)$. And suppose that $f$ is differentiable at $x_0$. Then $f'(x_0)=0$.
Mathematical analysis. Calculus.
#MathematicalAnalysis #Calculus
The same video on YouTube:
https://youtu.be/F0YBuDCV2Jc
The same video on Telegram:
https://t.me/mathematical_bunker/101
Loading comments...
-
 1:33:39
1:33:39
Redacted News
5 hours agoBREAKING! Europe goes NUCLEAR against Trump over pushing for PEACE in Ukraine | Redacted
117K156 -
 LIVE
LIVE
Melonie Mac
6 hours agoGo Boom Live Ep 38!
280 watching -
 1:41:00
1:41:00
Darkhorse Podcast
7 hours agoIf Only We’d Known: The 265th Evolutionary Lens with Bret Weinstein and Heather Heying
76.7K31 -
 1:58:29
1:58:29
Conspiracy Pilled
3 days agoThe Vaccine Conversation (S5 - Ep17)
18.9K -
 11:22
11:22
Tundra Tactical
4 hours ago $1.24 earnedUSA vs Canada HOCKEY Fight: The Real PRIDE Fighting.
25.3K -
 54:43
54:43
LFA TV
1 day agoWhy Exposing Waste and Fraud Terrifies the Beltway | TRUMPET DAILY 2.19.25 7PM
22.7K2 -
 1:01:13
1:01:13
Candace Show Podcast
5 hours agoBlake Lively's BOMBSHELL Legal Filing | Candace Ep 149
93.5K112 -
 1:11:22
1:11:22
Vigilant News Network
8 hours agoElon Musk Shuts Down RFK Jr. Critics With One Powerful Statement | The Daily Dose
65.2K24 -
 1:12:23
1:12:23
Dad Dojo Podcast
20 hours ago $1.15 earnedEP20: The Super Bowl and Solving The Economy
35.6K -
 4:49
4:49
Tactical Advisor
3 days agoBest Small Handgun Optics | CH PWS Shot Show 2025
24.3K2