Premium Only Content
This video is only available to Rumble Premium subscribers. Subscribe to
enjoy exclusive content and ad-free viewing.
Blanchet's theorem | plane geometry | intermediate level
2 months ago
8
Science
mathematics
geometry
plane geometry
classical geometry
Euclidean geometry
bisector
altitude
perpendicular
angle
ceva's theorem
Episode 76.
Blanchet's theorem | plane geometry | intermediate level.
Branch of mathematics: plane geometry.
Difficulty level: intermediate.
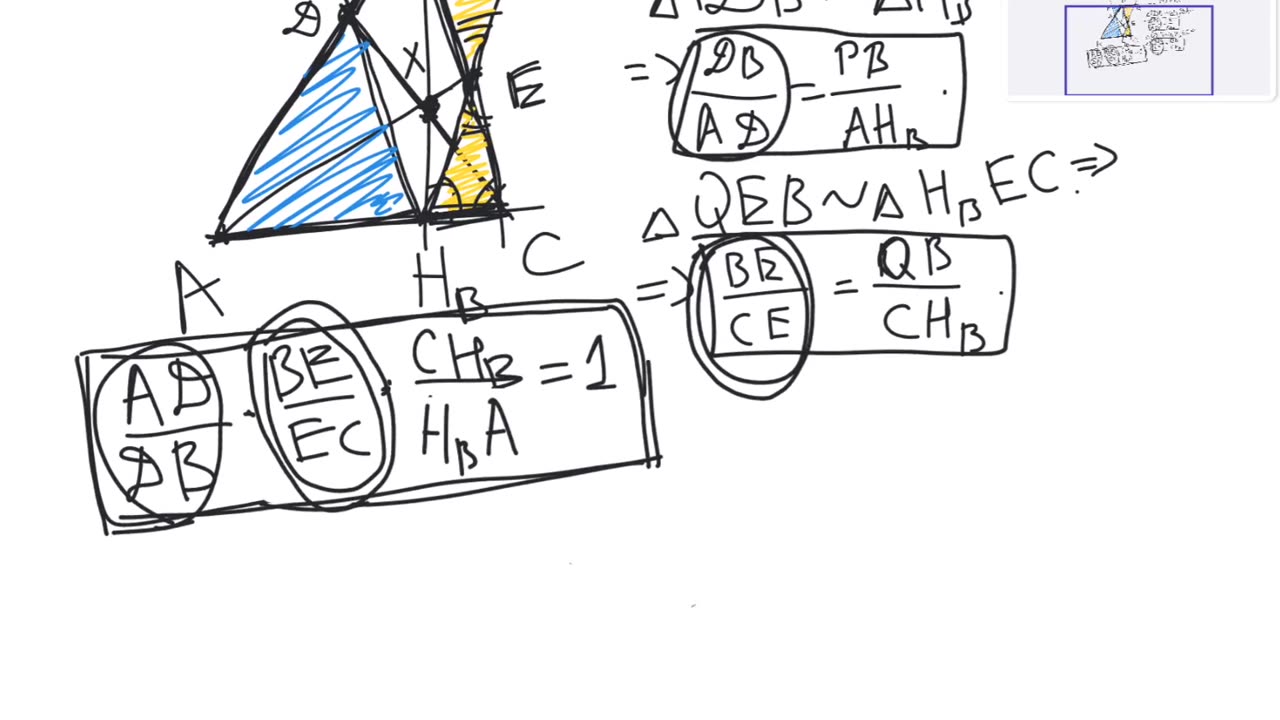
Theorem. Let $ABC$ be a triangle and let $BH_B$ be the altitude in this triangle. Let $X$ be an arbitrary point on the altitude $BH_B$. Let $D$ be the intersection point of $CX$ and $AB$. Let $E$ be the intersection point of $AX$ and $BC$. Then $H_BB$ is the bisector of the angle $DH_BE$ (in other words, $\angle{DH_BB}=\angle{EH_BB}$).
Mathematics. Geometry. Plane geometry.
#Mathematics #Geometry #PlaneGeometry
The same video on YouTube:
https://youtu.be/3H0DfsFqVOw
The same video on Telegram:
https://t.me/mathematical_bunker/100
Loading comments...
-
 42:24
42:24
MYLUNCHBREAK CHANNEL PAGE
20 hours agoA Century Gone
132K64 -
 38:22
38:22
Stephen Gardner
14 hours ago🔥HOLD ON! The RUMORS about Kamala are TRUE...
175K492 -
 1:22:44
1:22:44
Michael Franzese
1 day agoWill Trump’s Win Finally Convince Democrats to Stop The Woke Nonsense??
163K128 -
 8:27:07
8:27:07
MDGgamin
17 hours ago🔴LIVE- Rumble Gaming To The MOON - Variety of Games & Chatting - #RumbleTakeover
143K5 -
 27:24
27:24
Mr. Build It
5 days agoDECK DISASTER! How We Fixed a Botched Build
110K16 -
 26:58
26:58
barstoolsports
18 hours agoZach Bryan Blocks All of Barstool | Stool Scenes
121K17 -
 1:06:44
1:06:44
Talk Nerdy 2 Us
1 day ago🔥 Hackers vs. The World: From Amazon breaches to FBI-confirmed Chinese telecom spying
112K22 -
 1:24:20
1:24:20
Vigilant News Network
1 day agoJoe Rogan Drops Shocking Election Claim | The Daily Dose
174K182 -
 1:10:18
1:10:18
FamilyFriendlyGaming
1 day ago $19.38 earnedCat Quest III Episode 2
141K -
 20:07
20:07
DeVory Darkins
1 day ago $24.20 earned"They Talking About Finance!" The View FRUSTRATED by NYC Mayor TRUTH BOMB
106K123