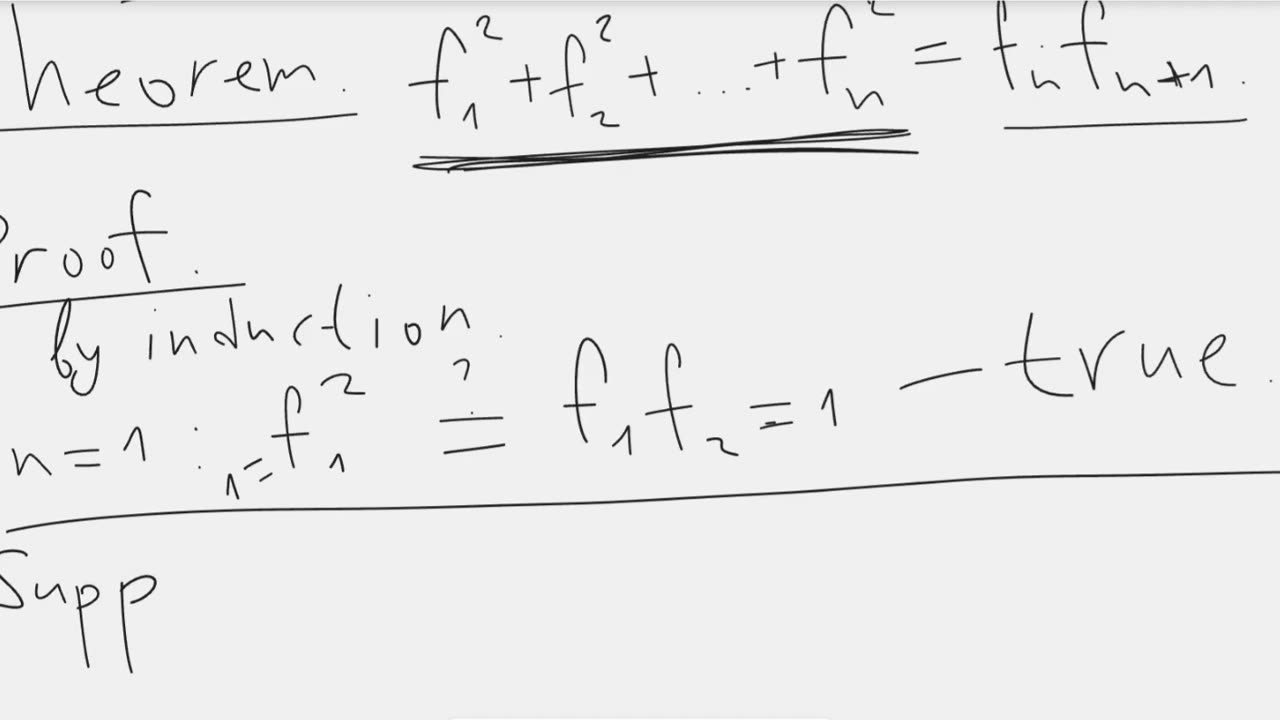
The sum of the squares of the first n Fibonacci numbers

6 months ago
27
Science mathematics fibonacci fibonacci numbers sum identity

Episode 30.

The sum of the squares of the first n Fibonacci numbers.
Theorem. The sum of the squares of the first n Fibonacci numbers is equal to the the product of n-th and (n+1)-th Fibonacci numbers. $f_1^2+f_2^2+f_3^2+\ldots+f_n^2=f_n f_{n+1}$.

The same video on YouTube:
https://youtu.be/cThQEuMDVvA

The same video on Telegram:
https://t.me/mathematical_bunker/54

Loading comments...