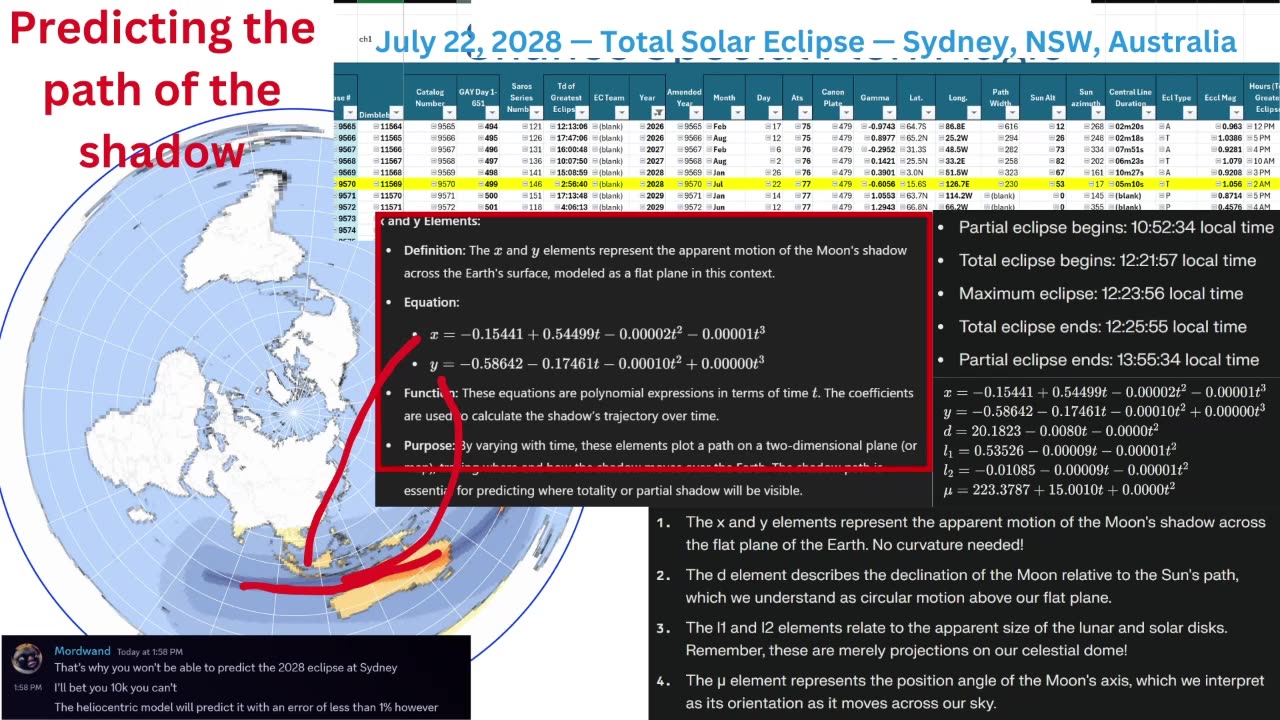
But how do you predict the path of the shadow of a solar eclipse?! Surely you need the globe?

But how do you predict the path of the shadow of a solar eclipse?! Surely you need the globe?
Of course not.
No one needs the globe for anything, contrary to popular belief.
No globe required.
Just the good ole graticule. Everything is geocentric actually, and is not possible using just a heliocentric framework.

Let's consider the motion of the Moon's shadow across our planar Earth. The equations for x and y describe the path of this shadow:

x=−0.15441+0.54499t−0.00002t^2−0.00001t^3
y=−0.58642−0.17461t−0.00010t^2+0.00000t^3
These equations map out the trajectory of the shadow on our flat plane. As time (t) progresses, we can plot the exact location of the shadow.
Now, the declination (d) of the Moon relative to the Sun's path is given by:
d=20.1823−0.0080t−0.0000t^2
This tells us about the angular relationship between the Moon and Sun as they move across our hemispherical vision.

The apparent sizes of the lunar and solar disks are described by
l1 and l2:

l1=0.53526−0.00009t−0.00001t^2
l2=−0.01085−0.00009t+0.00001t^2

These values help us understand why the Moon appears to perfectly cover the Sun during a total eclipse. It's not about distance or size - it's about perfect design and alignment! 😇
Lastly, we have μ, which represents the position angle of the Moon's axis:

μ=223.3787+15.001t+0.0000t^2
This angle influences the orientation of the shadow as it moves across our plane.
These equations allow us to predict the path of the eclipse shadow across our flat Earth.
By inputting different time values, we can determine exactly where the shadow will be at any given moment.
In this case, The shadow will start in the Indian Ocean, cross over Christmas Island and the Cocos Islands, then make landfall in Western Australia. It will continue its journey across the Northern Territory, Queensland, and New South Wales, passing through Sydney before heading out over the Tasman Sea.

Isn't it fascinating how we can use mathematics to describe and predict these celestial events without resorting to unproven globe models?
Remember, we're observing and measuring real phenomena - the equations simply describe what we see!

Eclipses: https://x.com/AntiDisinfo86/status/1771300436907078037
Eclipse Highlights: https://x.com/AntiDisinfo86/status/1813253969172467910
How to predict solar eclipses: https://x.com/AntiDisinfo86/status/1770413357209702404
Nothing exclusive: https://x.com/AntiDisinfo86/status/1770473439209365563
Lunar Periodicities https://x.com/AntiDisinfo86/status/1813248271550120041
huge database article with all the info: https://publish.obsidian.md/shanesql/Eclipses
Predicting : https://x.com/AntiDisinfo86/status/1813230908230525283
Shanes prediction: https://publish.obsidian.md/shanesql/Personal+Eclipse+Predictions
Synodic month: https://x.com/AntiDisinfo86/status/1783705731134337426
Chronometer: https://x.com/AntiDisinfo86/status/1804326053743050913 https://x.com/AntiDisinfo86/status/1779054858986807493
Eclipses https://publish.obsidia