Premium Only Content
Solar System Mars Size Comparison 2023 _ Mars _ Solar System
Data taken from Google:
Uranus;
The volume of Mars is 1.6318 × 10^11 cubic kilometers, and the volume of Uranus is 6.833 × 10^13 cubic kilometers. This means that you could fit 430.34 Marses inside of Uranus.
Please note that this is just a theoretical calculation, as Uranus is a gas giant and does not have a solid surface on which to place Marses.
Saturn;
Saturn is much larger than Mars, so you can fit many Mars-sized objects inside Saturn. To provide a rough estimate, let's compare their sizes:
The diameter of Mars is approximately 6,779 kilometers (4,212 miles), and the diameter of Saturn is approximately 116,464 kilometers (72,367 miles).
To calculate the number of Mars-sized objects that could fit inside Saturn, we can divide the volume of Saturn by the volume of Mars.
The volume of a sphere can be calculated using the formula: V = (4/3) * π * r^3, where "r" represents the radius of the sphere.
Let's assume the sizes of Mars and Saturn are proportional to their diameters. Therefore, the radius of Mars would be half of its diameter, and the radius of Saturn would be half of its diameter.
Calculating the volume of Mars:
V_mars = (4/3) * π * (r_mars)^3
= (4/3) * π * (6,779/2)^3
≈ 163,147,707,784 cubic kilometers
Calculating the volume of Saturn:
V_saturn = (4/3) * π * (r_saturn)^3
= (4/3) * π * (116,464/2)^3
≈ 827,129,375,406,853 cubic kilometers
Now, we can determine the number of Mars-sized objects that can fit inside Saturn:
Number of Mars-sized objects = V_saturn / V_mars
≈ 827,129,375,406,853 / 163,147,707,784
≈ 5,070
Therefore, approximately 5,070 Mars-sized objects can fit inside Saturn. Please note that this is a rough estimate, assuming the objects are perfect spheres and not considering variations in shape or surface irregularities.
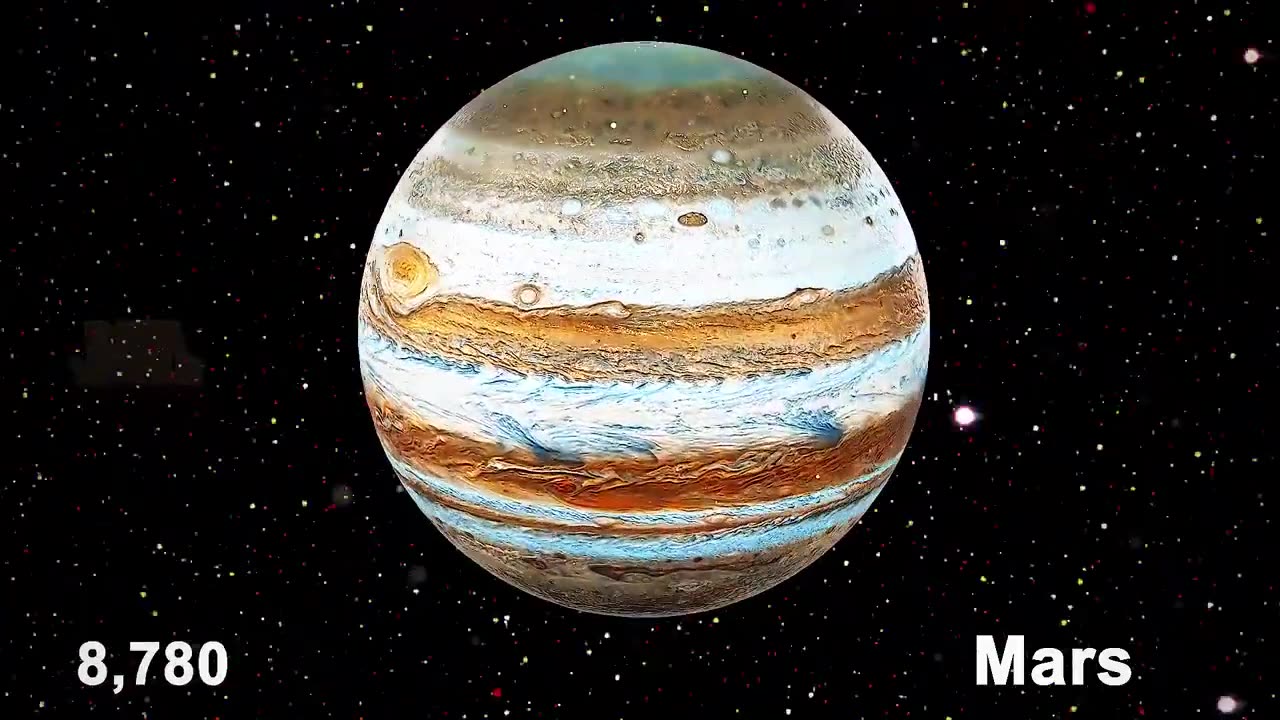
Jupiter;
Jupiter is the largest planet in our solar system, while Mars is significantly smaller. To give you an idea of the difference in size, let's compare their diameters.
The diameter of Jupiter is approximately 143,000 kilometers (88,800 miles), while the diameter of Mars is about 6,800 kilometers (4,200 miles).
To determine how many Mars-sized objects could fit inside Jupiter, we can divide the volume of Jupiter by the volume of Mars.
The volume of a sphere is given by the formula V = (4/3) * π * r^3, where r is the radius of the sphere.
Using the diameters provided earlier, we can calculate the volumes:
For Jupiter:
Radius = 143,000 km / 2 = 71,500 km
Volume = (4/3) * π * (71,500 km)^3 ≈ 1.43 x 10^15 cubic kilometers
For Mars:
Radius = 6,800 km / 2 = 3,400 km
Volume = (4/3) * π * (3,400 km)^3 ≈ 1.63 x 10^11 cubic kilometers
Now, dividing the volume of Jupiter by the volume of Mars:
1.43 x 10^15 cubic kilometers / 1.63 x 10^11 cubic kilometers ≈ 8,780
Therefore, approximately 8,780 Mars-sized objects could fit inside Jupiter.
-
 45:56
45:56
Glenn Greenwald
3 hours agoLee Fang Reacts to Trump's Speech to Congress; Will DOGE Tackle Military Waste? | SYSTEM UPDATE #418
21K35 -
 43:23
43:23
Donald Trump Jr.
4 hours agoNo Clap: Dems are a Disgrace but My Father is Bringing Back Common Sense | Triggered Ep.222
85.2K80 -
 LIVE
LIVE
Akademiks
4 hours agoDay 1/30. Drake Drops lawsuit vs iHeartMedia? Offset and Cardi Calls it Quits. 50 v Jim Jones?
3,037 watching -
 8:56:13
8:56:13
Dr Disrespect
10 hours ago🔴LIVE - DR DISRESPECT - WARZONE - 150 PLAYER LOBBIES
139K18 -
 1:27:35
1:27:35
Redacted News
4 hours ago"This is NOT normal" Trump just destroyed the woke mob as Dems in disarray | Redacted News Live
114K236 -
 1:39:52
1:39:52
Vigilant News Network
6 hours agoUNHINGED: Dems Say That Elon Needs to ‘Go Back to AFRICA?’ | The Daily Dose
84.9K18 -
 1:13:13
1:13:13
Sean Unpaved
5 hours ago $6.05 earnedQB Carousel
72.4K2 -
 1:04:28
1:04:28
Crypto Power Hour
23 hours ago $5.60 earnedThe Crypto Power Hour - ‘In Crypto We Trust’
65.7K5 -
 28:55
28:55
CatfishedOnline
5 hours agoWoman Love Bombed by Salt-and-Pepper Scammer
32.9K5 -
 14:51
14:51
Misha Petrov
4 hours agoDemocrats HUMILIATED at Trump’s Congressional Address! Breaking Down the MUST-SEE Moments
41.5K31