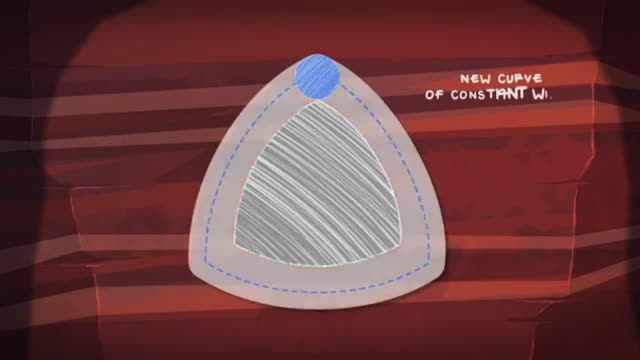
A circle has the largest edge.

For example, if you roll any curve of constant width around another,you'll make a third one.This collection of pointy curves fascinates mathematicians.They've given us Barbier's theorem,which says that the perimeter of any curve of constant width,not just a circle,equals pi times the diameter.Another theorem tells us that if you had a bunch of curves of constant width with the same width,they would all have the same perimeter,but the Reuleaux triangle would have the smallest area.The circle, which is effectively a Reuleaux polygon with an infinite number of sides,has the largest.