Premium Only Content
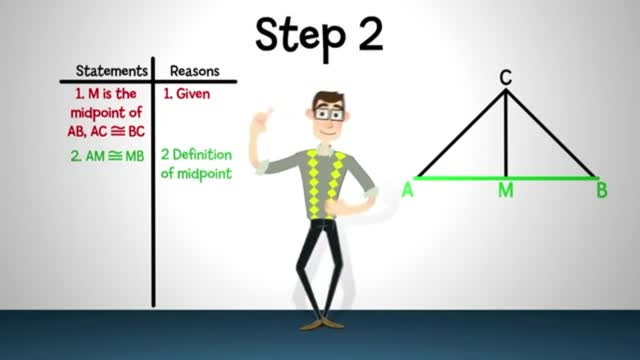
So how do we prove it?
So how do we prove it?First, I'll write down what we know.We know that point M is the midpoint of AB.We also know that sides AC and BC are already congruent.Now let's see. What does the midpoint tell us?Luckily, I know the definition of midpoint.It is basically the point in the middle.What this means is that AM and BM are the same length,since M is the exact middle of AB.In other words, the bottom side of each of our triangles are congruent.I'll put that as step two.Great! So far I have two pairs of sides that are congruent.The last one is easy.The third side of the left triangle is CM, and the third side of the right triangle is -well, also CM.They share the same side.Of course it's congruent to itself!This is called the reflexive property.
-
 3:16
3:16
Reuters
2 years agoExplainer: How to prove genocide?
2387 -
 0:18
0:18
TheMagicMatt
2 years agoProve me WRONG!😤#rockpaperscissors#interactive#trythis#versus
6062 -
 9:13
9:13
ericntunctu
2 years agoProve 3 by 3 determinant Krattenthaler's Identity
-
 58:10
58:10
Kimberly Guilfoyle
4 hours agoAmerica is Back & The Future is Bright: A Year in Review | Ep. 183
15.2K15 -
 3:03:27
3:03:27
vivafrei
9 hours agoEp. 242: Barnes is BACK AGAIN! Trump, Fani, J6, RFK, Chip Roy, USS Liberty AND MORE! Viva & Barnes
52.8K21 -
 LIVE
LIVE
Dr Disrespect
7 hours ago🔴LIVE - DR DISRESPECT - MARVEL RIVALS - GOLD VANGUARD
3,627 watching -
 1:15:00
1:15:00
Awaken With JP
5 hours agoMerry Christmas NOT Happy Holidays! Special - LIES Ep 71
75.8K81 -
 1:42:21
1:42:21
The Quartering
7 hours agoTrump To INVADE Mexico, Take Back Panama Canal Too! NYC Human Torch & Matt Gaetz Report Drops!
61.6K43 -
 2:23:15
2:23:15
Nerdrotic
6 hours ago $8.26 earnedA Very Merry Christmas | FNT Square Up - Nerdrotic Nooner 453
42.9K4 -
 1:14:05
1:14:05
Tucker Carlson
6 hours ago“I’ll Win With or Without You,” Teamsters Union President Reveals Kamala Harris’s Famous Last Words
122K270