Premium Only Content
Intuition behind Snell's Law
Let me help provide some intuition behind Snell's Law.
If you're not satisfied with just the intuition
I'll show in a follow-up video how the geometry works to get Snell's Law
First thing to understand with snell's law is what the index of refraction, n, is
n is basically a measure of how fast travels through a medium
Our baseline is n=1 for light traveling through space
If light traveled half that fast through a medium, the medium would have
n2=2
so the speed of light through the medium would be
v2=cn2
Or half the speed of light in a vacuum
Demo:
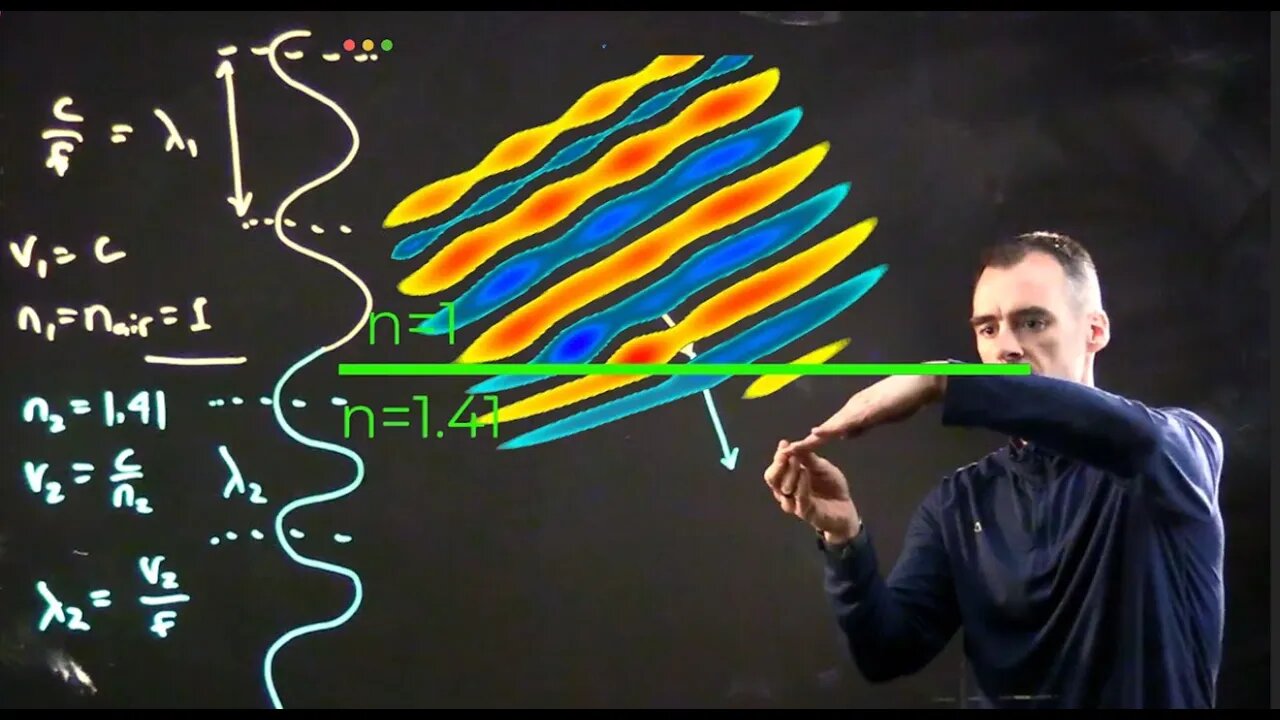
So lets see how this would work for a plane wave
So imagine we have a region with n=1 on top and n=2 in the gray region below the green line
The plane wave is starting up here, propagating downward
You can see when it hits this new medium, the wave slows down
It has the same frequency because the phase at the line has to match
But it has different wavelength
If the wavelength on top is lambda_1
Then the wavelength on bottom is
𝜆2=𝜆1∗n1n2
, or .71 lambda_1 in this case
Now imagine if this plane wave was incident at an angle
The part of the wavefront that hits the medium first slows down
While the part that hasn't hit it yet keeps going at normal speed
The side slowing down causes the wavefront angle to shift
And this wave front is perpendicular to the direction the beam is traveling
So you can see up here the wavefront is like this,
And propagation is perpendicular
Now here the wavefront angle shifts since one side slowed down first
And the propagation direction is perpendicular, so it also shift
This gives rise to Snell's law, which states:
n_1sin(\theta_1)=n_2sin(\theta_2)
Where n_1 and n_2 are the refractive indecis' as discussed
And theta_1 and theta_2 are the angles with respect to the normal line to the interface of the mediums
So this is the basic intuition of how it works. If you're not completely satisfied with the inuition and want to really understand where this equation comes from, I'll show that in the next video. It's not that bad, just need a little geometry to show how we get this.
-
 1:56:25
1:56:25
Nerdrotic
12 hours ago $16.45 earnedThe Red Pyramid's Hidden Secrets | Forbidden Frontier #091
60.3K13 -
 2:08:53
2:08:53
vivafrei
20 hours agoEp. 252: Liberals DISQUALIFY Candidate from Race! DOGE Wins & Loses; Rumble Sues BRAZIL! & MORE!
148K250 -
 1:15:12
1:15:12
Josh Pate's College Football Show
11 hours ago $21.27 earnedCFB’s Top 12 Programs | TV Executives & Our Sport | USC Changes Coming | Early Championship Picks
88.1K2 -
 LIVE
LIVE
Vigilant News Network
15 hours agoUK Government BUSTED in Secret Plot to Extract Your Data | Media Blackout
1,702 watching -
 1:03:32
1:03:32
Winston Marshall
3 days ago"War On Children!" The DEMISE Of The West Starts With Schools - Katharine Birbalsingh
123K67 -
 48:02
48:02
Survive History
18 hours ago $8.75 earnedCould You Survive as a Sharpshooter in the Napoleonic Wars?
72.7K3 -
 12:03
12:03
Space Ice
19 hours agoSteven Seagal's China Salesman - Mike Tyson Knocks Him Out - Worst Movie Ever
54K19 -
 11:37
11:37
Degenerate Jay
18 hours ago $19.35 earnedJames Bond Needs Quality Over Quantity From Amazon
120K11 -
 15:23
15:23
Misha Petrov
18 hours agoTrad Wives & Girl Bosses Go to WAR!
87.2K52 -
 2:03:11
2:03:11
TheDozenPodcast
17 hours agoFootball villain fighting the state: Joey Barton
66.4K1